|
Feature: Calculating Pi
Posted by Nick on 14 March 2000, 03:57 GMT
I hope everyone is having a very happy Pi Day! In case you don't know, today (March 14) is Pi Day. In honor of this, this week's feature will be in homage of our favorite irrational number. Andy Selle has written us a feature regarding pi and its origins. Check out the updated version instead. It is available in PDF or HTML
Calculating  : an explorative tour : an explorative tour Andrew Selle (aselle@ticalc.org)
Abstract: A look at calculating 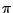 with a TI graphing calculator. This article goes through a variety of methods for finding the ever closer decimal approximation of our favorite trancendental number.
Introduction 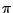 is a very interesting number. We are first introduced to it early in our grade-schooling as a component of the formulas is a very interesting number. We are first introduced to it early in our grade-schooling as a component of the formulas  and and 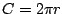 . It seems odd to us that we need to use this number. Where did it come from? How did we ever figure it out? These our questions that I hope to address and perhaps answer. However, the major point of this article is to explore methods of calculating . It seems odd to us that we need to use this number. Where did it come from? How did we ever figure it out? These our questions that I hope to address and perhaps answer. However, the major point of this article is to explore methods of calculating 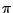 using a Texas Instruments graphing calculator (and perhaps a few computers). using a Texas Instruments graphing calculator (and perhaps a few computers).
History The history of 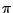 is a long one. The first significant advancement in the understanding of is a long one. The first significant advancement in the understanding of 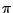 was the Ancient Greeks. They came up with the concept of inscribing a polygon inside a circle. As you added more sides to the polygon, the polygon became closer and closer to a circle. By taking the ratio between the polygon's area to the circle's area, you could find was the Ancient Greeks. They came up with the concept of inscribing a polygon inside a circle. As you added more sides to the polygon, the polygon became closer and closer to a circle. By taking the ratio between the polygon's area to the circle's area, you could find 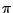 Thus, the first method of calculating Thus, the first method of calculating 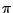 was born. was born.
During the middle ages, time for calculating 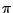 was not readily available. Even when it was, the polygon inscribing method was very slow and painstaking. It was eventually discovered that an infinite series approximating was not readily available. Even when it was, the polygon inscribing method was very slow and painstaking. It was eventually discovered that an infinite series approximating 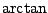 was a good means to calculate was a good means to calculate 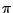 . Once the computer was invented, . Once the computer was invented, 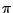 was quickly calculated to many places. was quickly calculated to many places.
This history is in no way complete, but it covers the major ideas.
Math Obviously, a lot of math is involved in calculating 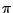 . I will derive all my mathematics as completely as possible, but don't be afraid to skip the derivations if you are not interested. I will highlight the important results found to make this easy. . I will derive all my mathematics as completely as possible, but don't be afraid to skip the derivations if you are not interested. I will highlight the important results found to make this easy. Monte Carlo Method Derivation One of my favorite methods, and one of the simplest to understand is the Monte Carlo method. I first ran into this method when I was learning BASIC for my Apple II. One sets up a square that is 2 by 2. One then inscribes a circle inside the square. Random points are then plotted repeatedly. For each point we add one to the variable n, and if the point is within the circle we add one to the variable i. Then to calculate we simply put together what we know. 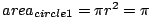 ,areasquare = 4. Now, we can setup the proportion ,areasquare = 4. Now, we can setup the proportion 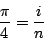 . Thus, . Thus,  Implementation This program can be easily implemented in 8x TI-BASIC as: :0 --> N0 --> D
:While 1
:rand*2-1 --> X
:rand*2-1 --> Y
:If sqrt(x^2+y^2)=< 1
:N+1 --> N
:End
:D+1 --> D
:Disp (N/D*4)
:End
Bibliography 1) Dara Hazeghi: Dara's Pi Page http://www.geocities.com/EnchantedForest/5815/,
Accessed 3/4/2000
2) JOC/EFR: Pi Through the Ages http://www-groups.dcs.st-and.ac.uk/
If you don't yet know how to celebrate Pi Day, here's a few tips: - Watch the movie Pi. It was written rather recently, and it's a bit artsy, but I love it. I'm planning on watching it with friends during the evening.
- Serve pie to your classes, or if you don't have enough pie, have it for dinner!
- Be psychotic like me and memorize sixty digits of pi. :)
Best wishes to you during this Pi Day season.
|
|
|
|
|
The comments below are written by ticalc.org visitors. Their views are not necessarily those of ticalc.org, and ticalc.org takes no responsibility for their content.
|
|
|
Re: Feature: Calculating Pi
|
Gohan 
(Web Page)
|
|
Pi=3.14, nothing really to get so excited about. (in my opinion) LoL!
|
|
|
14 March 2000, 05:13 GMT
|
|
|
Re: Feature: Calculating Pi
|
Mike Fletcher 
(Web Page)
|
|
On PI day '99 I found the poem at http://users.aol.com/s6sj7gt/mikerav.htm
It's called "Near a Raven" and it's a constricted poem about PI modeled after Poe, it's something to read with friends on Pi day!
Mike
|
|
|
14 March 2000, 05:16 GMT
|
|
|
Re: Feature: Calculating Pi
|
|
RongZeng
|
|
So...Did anyone have any pi?
|
|
|
14 March 2000, 05:48 GMT
|
|
|
HELLO PEOPLE!!!!
|
BrandonAGR 
(Web Page)
|
|
Jeez you people, do you not even read the article! The code that he presented will not work. The formula is wrong.
I modified the program above to make it better, so here it is. It is written in Ti-83 Basic.
:ClrDraw
:0->N:0->D
:-1->Xmin:1->Xmax
:-1->Ymin:1->Ymax
:Circle(0,0,1
:While 1
:rand*2-1->X
:rand*2-1->Y
:Pt-On(X,Y
:If sqrt(X^2+Y^2)<=1
:N+1->N
:D+1->D
:Text(0,0,(4N/D)
:End
And I don't think that this method is that good, it only gives pi after it runs for like 3 hours.
|
|
|
14 March 2000, 06:03 GMT
|
|
1 2 3 4 5 6 7
You can change the number of comments per page in Account Preferences.
|

